 |
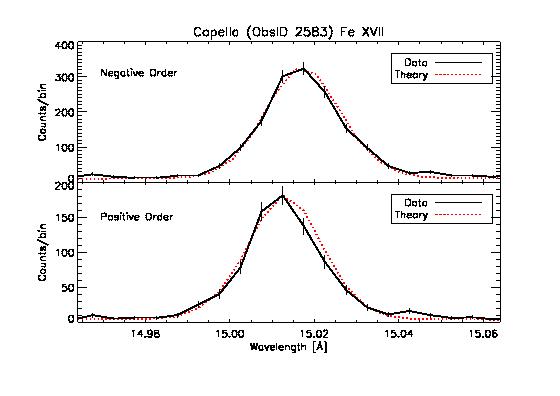
| FIGURE 6: Capella MEG + ACIS-S profiles for the Fe XVII 15.01 Å resonance line, in both plus and minus orders (solid black), with theoretical profiles overplotted (dashed red). |
Unless, of course, the inquisitor is an expert, such as a Chandra Users Committee member, a taxi driver, or a pizza delivery person. In this case one might point out before heading to the bathroom that, among all the other aspects of the Chandra spectrographs that are rather difficult to calibrate, the instrumental profile, or "line response function" (LRF) as it is referred to, is perhaps about the trickiest bit. At the X-ray Calibration Facility (XRCF) at Marshall Space Flight Center, many tests of the imaging characteristics of the instrumentation were devised and carried out. However, on the ground the primary mirrors were distorted under their own weight, and the electron impact X-ray sources were not quite points. The ground-based testing could only verify complicated models of the mirror and detector imaging performance including the gravitational distortion. The true performance of the system was only revealed later, in orbit.
Once flying, X-ray point sources can, in principle, provide the point spread function in exquisite detail. While the realities of effective area and detector performance tend to mock such principles - pile-up effects in ACIS mean that fainter sources are best, but these require long exposure times - the LRF is only onedimensional and so should be easier. All that is needed is a bright point source with narrow, isolated lines spaced every couple of Ångstrom or so between 1.2 and 170 Å. At this point, something strong to wash down the rich cheese and spinach nibbly things is a good idea.
Our best hope of bright, narrow lines in a cosmic point source is in the hot coronae of stars. In these plasmas, non-thermal velocities are hopefully rather small and, provided we choose a source with a small v sin i, intrinsic source line profiles should be dominated by thermal (Gaussian) broadening. However, atoms of Fe at a temperature of 10 million K have a typical velocity of about 50 km s-1, corresponding to roughly 1/5 of the instrumental line width at a typical Chandra grating resolving power of 1000 - small but sufficiently large that it must be taken into account when trying to understand the instrumental broadening component. Useful lines of H-like ions are also broadened by their doublet splitting: the O VIII 19 Å Lyman α doublet splitting is 0.0055 Å, for example, or about 1/3 of a line width - small, but sufficiently large that it justifies another stiff drink while pondering the complications.
Like line blends that inevitably distort the wings of spectral line profiles in even the best diagnostic lines that have been carefully selected by model calculations to be relatively blendfree ..... Close examination of high quality spectra of even the most well-behaved sources, such as the Chandra grating calibration target Capella (G1 III + G8 III), will show that there is no single line whose profile remains untainted down to less than 90% or so of peak intensity. This is almost guaranteed by the pseudo-continuum of Fe and Ni "L-shells" for wavelengths less than 18 Å or so, and at longer wavelengths in the LETG bandpass by analogous transitions in other abundant lighter elements Ne-Ar.
Another problem is simply that of photon statistics. Placing the diffraction gratings behind the mirrors produces a sometimes disappointingly small slice of effective area for the first orders. The Chandra mirrors are also very slightly asymmetrically aligned and this is reflected in spectral line profiles; to do the calibration properly requires looking at positive and negative orders separately. From the point of view of the source, we are interested in photons from only a few bright lines that cannot be easily coadded because the thermal and non-thermal line broadening contributions at different wavelengths and for lines of different species affect the observed profile to different extents.
Now that a firm foundation of excuses is in place, and since a large crowd of eager eavesdroppers is now hanging on every syllable (that looks like Beyoncé over there), how is the calibration of the spectrograph instrumental profile going? Like methods used to calibrate the PSF, the LRF calibration involves more a testing of model predictions, rather than measurement of a quantity that is then used to derive the calibration product. Coincidently, this approach also has the striking advantage of being much easier - innocent until proven guilty of calibration.
 |
| FIGURE 6: Capella MEG + ACIS-S profiles for the Fe XVII 15.01 Å resonance line, in both plus and minus orders (solid black), with theoretical profiles overplotted (dashed red). |

We can also compare the widths at half maximum intensity of observed and synthetic line profiles. The line FWHM is less sensitive to low-level blends than the full line profile, and we can measure it quite accurately in both observed and synthetic line profiles by fitting functions such as that in Equation 1. Line widths were derived in this way in a recent paper by Chung et al. (2004) for Capella and also for Algol (B8V + K2 IV; Porb= 2.87d). While line widths for Capella were found to be fairly consistent with synthetic profiles, those observed in Algol seem systematically too large and indicate that extra broadening in the source is at work - either a radially extended corona or nonthermal plasma motion (Figure 7).
"Now, Britney, did I tell you about the time when the Project Scientist..."
Analyze That
One of the most spectacular LETGS observations of the
year must be that of the nova V4743 Sgr. It became the brightest X-ray
source in the sky at wavelengths above 25 Å (< 0.5 keV) in early 2003,
and was caught for 25 ks by the LETGS as a target of opportunity
(P.I. S. Starrfield). A preliminary analysis of its spectrum (Figure
8) was presented by Ness et al. (2003). While prominent resonance
lines of C, N and O can easily be identified, unlike the case of
low-density plasmas, the higher density environment of the nova
atmosphere in the gravity of its degenerate host can support
substantial absorption from excited states that are more difficult to
identify. Determining the origin of
the multitude of weaker lines gouging the continuum presents a
considerable challenge.
Observer and proposer information and news on the performance of the Chandra LETGS can be found on the instruments and calibration page: http://cxc.harvard.edu/cal/Links/Letg/User/
Jeremy Drake
References
Chung, S., Drake, J.J., Kashyap, V.L., Lin, L., Ratzlaff, P.W., ApJ, in press
Ness, J.-U. et al. 2003, ApJL, 594, L127